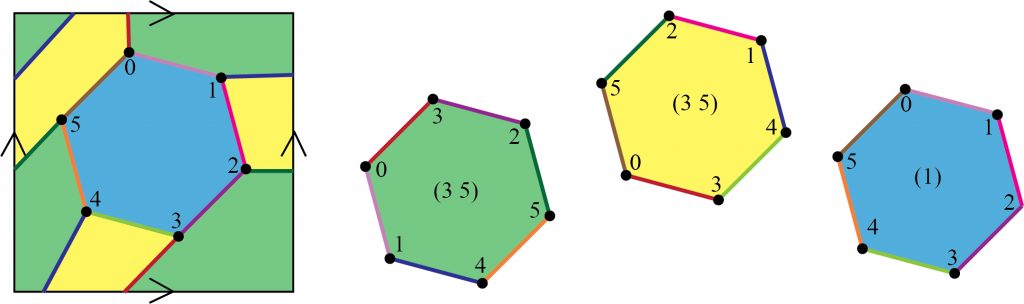
Home » Embedding Complete Bipartite Graphs with Cayley Maps » K3,3

K3,3

The optimal genus for $K_{3,3}$ is $g=1$ . We were able to obtain the same genus using a Cayley Map with group $Z_6$ and rotation $\rho=(1$ $3$ $5)$ .

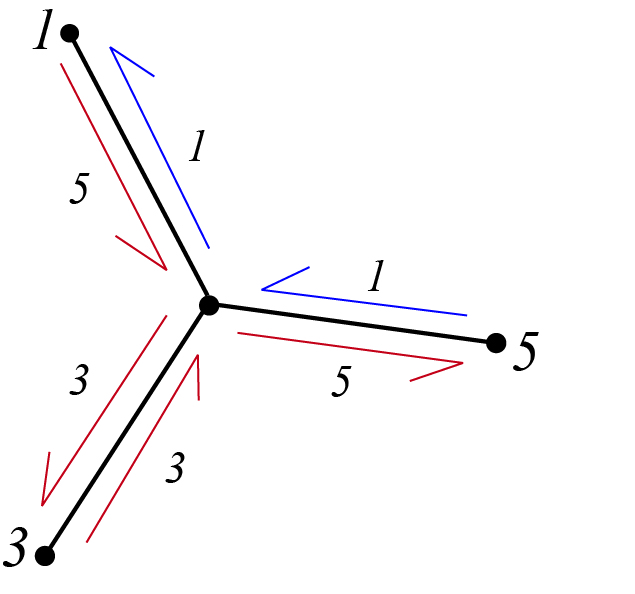
This image has an empty alt attribute; its file name is k33.jpg — $C_M(Z_6,($ 1 3 5 $))$
The face set is $\mathcal{F}=\{($ 3 5 $),($ 1 $)\}$ , where $F_0$ and $F_1$ are both 6-gons, and there are two $F_0$ faces. Using the Euler Characteristic Formula, $\chi=0$ , so g=1.

$C_M(Z_6,($ 1 3 5 $))$
The face set is $\mathcal{F}=\{($ 3 5 $),($ 1 $)\}$ , where $F_0$ and $F_1$ are both 6-gons, and there are two $F_0$ faces. Using the Euler Characteristic Formula, $\chi=0$ , so g=1.

Cayley Map $C_M(K_6,(1$ $3$ $5))$ embedded into a torus ( $g=1$ ). When the vertices are connected, the three hexagons *(right)* form a torus *(left)*. The green and yellow faces use the permutation $\lambda_0=(3$ $5)$ , and the blue face uses the permutation $\lambda_1=(1)$ .

Cayley Map $C_M(K_6,(1$ $3$ $5))$ embedded into a torus ( $g=1$ ). When the vertices are connected, the three hexagons *(right)* form a torus *(left)*. The green and yellow faces use the permutation $\lambda_0=(3$ $5)$ , and the blue face uses the permutation $\lambda_1=(1)$ .

Because the rotation for $Z_6$ only has three elements, there is only one other possible rotation, $\rho=(3$ 1 $5)$ . This rotation produces the same genus as $\rho=(1$ $3$ $5)$ , but with different faces:

*** QuickLaTeX cannot compile formula:
\mathcal{F}={(3

*** Error message:
Missing } inserted.
leading text: $\mathcal{F}={(3$

*** QuickLaTeX cannot compile formula:
1),(5)}

*** Error message:
Extra }, or forgotten $.
leading text: $1),(5)}

. Using $D_3$ instead of $Z_6$ produces the same genus as well, but $\mathcal{F}={\rho}$ .

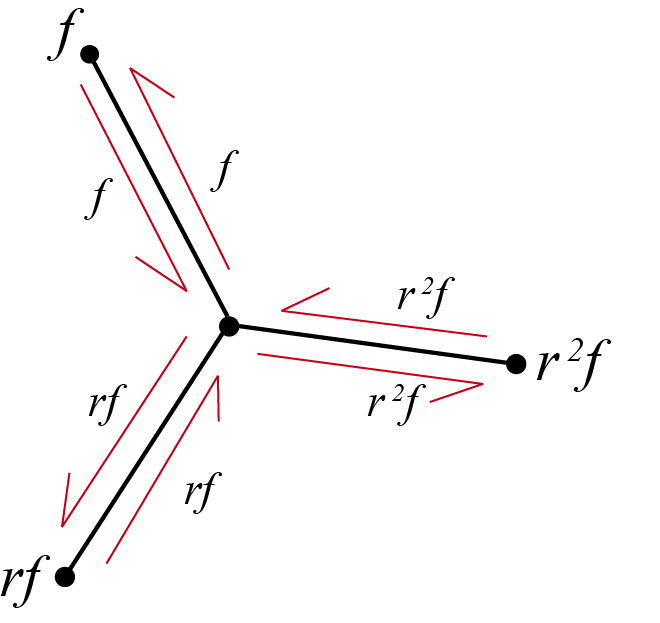
This image has an empty alt attribute; its file name is k33D3.jpg — $C_M(D_3,($ frfr^2f))\mathcal{F}=\{(f
*** QuickLaTeX cannot compile formula: \hspace{1} *** Error message: Illegal unit of measure (pt inserted). leading text: $\hspace{1}
rf
*** QuickLaTeX cannot compile formula: \hspace{1} *** Error message: Illegal unit of measure (pt inserted). leading text: $\hspace{1}
r^2f)\} $, and$ \lambda_0 $produces three 6-gons. Using the Euler Characteristic Formula,$ \chi=0 $, so$ g=1$.

$C_M(D_3,($ frfr^2f))\mathcal{F}=\{(f
*** QuickLaTeX cannot compile formula: \hspace{1} *** Error message: Illegal unit of measure (pt inserted). leading text: $\hspace{1}
rf
*** QuickLaTeX cannot compile formula: \hspace{1} *** Error message: Illegal unit of measure (pt inserted). leading text: $\hspace{1}
r^2f)\} $, and$ \lambda_0 $produces three 6-gons. Using the Euler Characteristic Formula,$ \chi=0 $, so$ g=1$.

Graph Embeddings

Menu

K3,3